The figure below shows a DVD in its rectangular storage case.
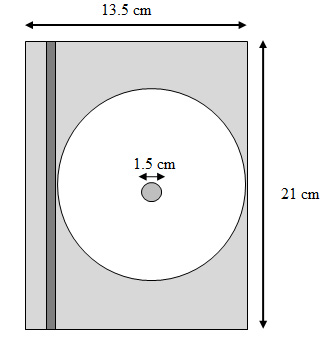
Which is the closest to the area of the storage case not occupied by the DVD if the DVD has a diameter of 12 centimeters?
- 170.4 cm2Incorrect. Add in the area for the center circle in the DVD.
- 163.3 cm2Incorrect. The small circle in the center of the DVD is not occupied by the DVD as well.
- 172.2 cm2Correct! you successfully identified the regions of the case not occupied by the DVD.
- 283.5 cm2Incorrect. This is the area of the rectangular case, but you need to remove the area of the DVD from this measurement.
The figure below shows a triangle on top of a rectangle.
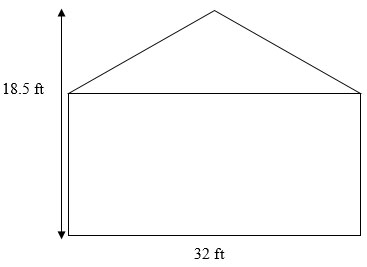
If the area of the triangle is 96 square feet, which of the following represents the area of the rectangle?
- 400 ft2Correct!
- 592 ft2Incorrect. It appears you multiplied the base and height of the entire figure.
- 496 ft2Incorrect. It appears you multiplied the base and height of the entire figure.and then subtracted the area of the triangle.
- 296 ft2Incorrect. It appears you used the wrong formula.
A triangle is to be cut out of a square piece of paper that measures 12 inches on each side, as shown below.
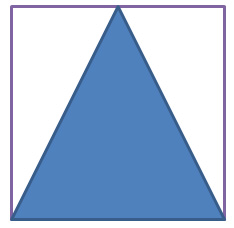
What is the amount of paper left over after the triangle is cut out?
- 144 in2Incorrect. Don’t forget to subtract the area of the triangle.
- 72 in2Correct! You found the difference of the areas of the square and the triangle.
- 108 in2Incorrect. Check your area formulas for the triangle and the square (you may need to use a rectangle formula for the square).
- 216 in2Incorrect. Once you have the areas of the triangle and the square, what operation do you need to do if the triangle is cut out of the square?
What is the area of the unshaded part of the rectangle below? You may assume that angles appearing to be right angles are right angles.

- 8800 ft2Incorrect. You found the area of the larger rectangle. Now you need to remove the areas of the shaded regions.
- 8200 ft2Incorrect. You also need to subtract the area of the shaded rectangle.
- 6400 ft2Incorrect. You also need to subtract the area of the shaded triangle.
- 5800 ft2Correct! You successfully found the area of the larger rectangle then subtracted the areas of both the triangle and the smaller rectangle.
The circle is going to be cut out of the rectangle as shown below.
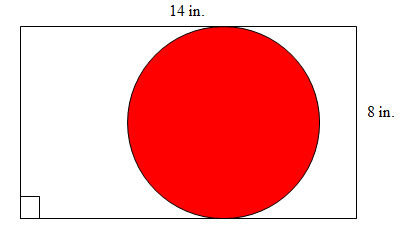
What is the best estimate of the area of the rectangle left over after the circle is cut out?
- 112 in2Incorrect. You found the area of the rectangle. Now you need to remove the areas of the shaded region.
- 62 in2Correct! You have successfully found the difference between the area of the rectangle and the circle.
- 313 in2Incorrect. The length of the rectangle is equal to the diameter of the circle, not its radius.
- 99 in2Incorrect. The area formula for a circle requires you to square the radius.





