In this lesson, we are going to take a close look at trapezoids and kites. Let’s start with a focus on the kite.

Open this applet, http://www.mathopenref.com/kite.html:

1. Make sure the box “Show diagonals” is checked.
2. Move each point around individually to observe the kite.

In your notes, answer the questions below. You can check your answers by clicking on each blank.

1. Describe the angles. 
   
   Interactive popup. Assistance may be required. Check Your Answer Two of the opposite angles are always congruent.
   
   
2. Are the non-vertex angles ∠BCD and ∠BAD always congruent?
   
   Interactive popup. Assistance may be required. Check Your Answer No, they are never congruent.
   
   
3. Are the vertex angles ∠ABC and ∠ADC always congruent?
   
   Interactive popup. Assistance may be required. Check Your Answer Yes, they are always congruent.
   
   
4. Explain what happens as you change the size of the kite.
   
   Interactive popup. Assistance may be required. Check Your Answer As you change the size of the kite, the diagonals are always perpendicular and the two angles that are not vertex angles are always congruent.
   
   
5. Complete the Kite Angles Conjecture:
   
   The Interactive button. Assistance may be required. _____ nonvertex angles of a kite are Interactive button. Assistance may be required. _____ congruent.
   
   
   
6. Describe the diagonals.
   
   Interactive popup. Assistance may be required. Check Your Answer The vertex angles are bisected by the diagonal.
   
   
7. Complete the conjecture about the diagonals of a kite:
   
   The diagonals of a kite are Interactive button. Assistance may be required. _____ perpendicular.
   
   
8. Observe the two diagonals. Describe their relationship. 
   
   Interactive popup. Assistance may be required. Check Your Answer The diagonal that intersects the vertex angles also bisects the diagonal that intersects the nonvertex angles.
   
   
9. Do either of the diagonals bisect the other? When the shape of the kite is changed does this observation stay true?
   
   Interactive button. Assistance may be required. _____ Yes, when you change the shape of the kite, the diagonal that intersects the vertex angles also bisects the diagonal that intersects the nonvertex angles.
   
   
10. Complete the conjecture about the diagonal connecting the vertex angles of a kite:
    
    The diagonal connecting the vertex angles of kite Interactive button. Assistance may be required. _____ bisects the diagonal between the nonvertex angles.
    
    
11. Do either of the diagonals bisect any of the angles? Does this property stay the same when you change the shape of the kite by dragging one of the vertex angles? Explain your observations. Interactive button. Assistance may be required.
    
    Interactive popup. Assistance may be required. Check Your Answer A diagonal bisects the vertex angles of the kite. The nonvertex angles of the kite are congruent but they are not bisected by a diagonal.
    
    
12. Complete the conjecture about the vertex angles of a kite:
    
    The Interactive button. Assistance may be required. _____ vertex angles of a kite are Interactive button. Assistance may be required. _____ bisected by a Interactive button. Assistance may be required. _____ diagonal.

Good going! Let's add kites to our quadrilateral flow chart.

Time for our last quadrilateral! Let's talk about trapezoids.

A trapezoid is a quadrilateral with exactly one pair of parallel sides. The parallel sides are called the bases of the trapezoid. The nonparallel sides are called legs of the trapezoid. In this figure, AB and CD are the bases of the trapezoid.

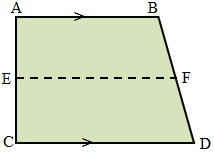
Base angles of a trapezoid are pairs of angles whose common sides are a base of the trapezoid.

∠A and ∠B are base angles and ∠C and ∠D are base angles of trapezoid ABCD. The segment that joins the midpoint of the two legs is called the midsegment of the trapezoid. EF is the midsegment of the figure above.

Let's investigate some relationships involving the midsegment of a trapezoid.

Open this applet http://www.mathopenref.com/trapezoidmedian.html, and move each point around individually to observe the trapezoid.

In your notes, answer the following questions:

1. Compare and contrast the two bases and the median. 
   
   Interactive popup. Assistance may be required. Check Your Answer The two bases and the median different lengths and they are parallel to each other.
   
   
2. Compare the lengths of the bases and the median.
   
   Interactive popup. Assistance may be required. Check Your Answer The median is half the length of the sum of the two bases.
   
   
3. Formulate the Trapezoid Midsegment Conjecture: The midsegment of a trapezoid is _____ to the bases and is equal in length to _____.
   
   Interactive popup. Assistance may be required. Check Your Answer The midsegment of a trapezoid is parallel to the bases and is equal in length to half the length of the sum of the bases.

Trapezoid Midsegment Theorem

The midsegment of a trapezoid is parallel to each base of the trapezoid and it's length is one-half the sum of the lengths of the two bases.

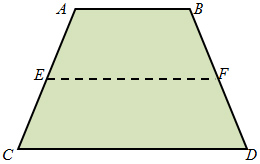
This theorem is telling us that EF || AB and EF || CD.

It also tells us that EF = AB + CD 2

Another way to write the formula for finding EF is 2(EF) = AB + CD.

For Example

Let's move on to Isosceles Trapezoids.

A special trapezoid is one in which the legs of the trapezoid are congruent. Trapezoid ABCD at the right is an isosceles trapezoid since the two legs, AC and BD are congruent.

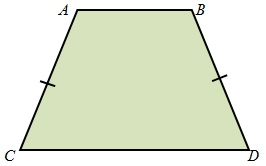
Let's look at a few facts about an isosceles trapezoid.

For Trapezoid ABCD above, this means that ∠A ≅ ∠B and ∠C ≅ ∠D.

The converse of this theorem, Trapezoid Theorem #2, provides a way to prove that a trapezoid is an isosceles trapezoid.

With this information, if you know that Quadrilateral TRAP is a trapezoid and you know that ∠P ≅ ∠A, then we also know TRAP is an isosceles trapezoid.

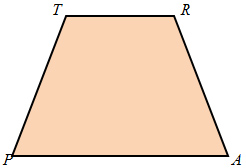
Now for our last isosceles trapezoid theorem.

Let's add the trapezoid information to our quadrilateral flow chart.

Pretty cool!

Now that you have completed the quadrilateral flow chart, click here for a printable chart. Print it out so that you have it with you in your geometry notebook.